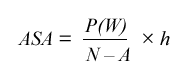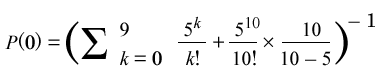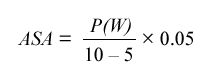What is Erlang C Formula?
The Erlang C Formula is a mathematical equation used to calculate the number of agents needed in a contact center, given the number of calls and the desired or target service level. It helps determine the probability that a call will wait in a queue, considering the traffic intensity (A) and the number of available agents.How to Calculate Erlang C Formula
The Erlang C formula is used to calculate the probability that a call will wait in a queue, given the traffic intensity and the number of agents available. Here are the steps to calculate it:- Calculate Traffic Intensity (A): A = λ × h where:
- (\lambda) = average number of calls per time period
- (h) = average handling time per call
- Calculate the Probability of Zero Calls in the System (P(0)):
where:- (N) = number of agents
- Calculate the Probability that a Call Waits (P(W)):
- Calculate the Average Speed of Answer (ASA):
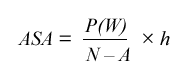
Example Calculation
Let's say a call center receives 100 calls per hour ((\lambda = 100)) and the average handling time is 3 minutes ((h = 3/60 = 0.05) hours). If there are 10 agents ((N = 10)):- Traffic Intensity (A): A = 100 x 0.05 = 5
- Probability of Zero Calls in the System (P(0)):
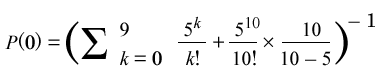
- Probability that a Call Waits (P(W)):
- Average Speed of Answer (ASA):
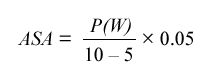
Why Erlang C Formula Matters
The Erlang C Formula is crucial for contact centers as it helps in:- Optimizing Staffing Levels: Ensuring the right number of agents are scheduled to meet call demand.
- Improving Customer Satisfaction: Reducing wait times and improving service levels.
- Cost Management: Balancing the cost of staffing with the need to provide timely service.
Factors Influencing Erlang C Formula
Several factors can influence the effectiveness of the Erlang C Formula:- Call Volume: The number of incoming calls.
- Average Handling Time (AHT): The time taken to handle each call.
- Agent Availability: The number of agents available to take calls.
- Service Level Targets: The desired percentage of calls answered within a specific time frame.
Strategies to Improve Erlang C Formula
To enhance the accuracy and effectiveness of the Erlang C Formula, consider the following strategies:- Accurate Forecasting: Use historical data to predict call volumes accurately.
- Flexible Staffing: Implement flexible staffing schedules to handle peak call times.
- Training and Development: Improve agent efficiency through training and development programs.
- Technology Integration: Use advanced call routing and workforce management software to optimize scheduling.
Related Terms
- Erlang B Formula: Used to calculate the number of lines required in a system without queuing.
- Service Level Agreement (SLA): A commitment between a service provider and a client regarding service standards.
- Average Speed of Answer (ASA): The average time taken to answer a call.
Conclusion
The Erlang C Formula is a vital tool for contact centers to optimize staffing levels, improve customer satisfaction, and manage costs effectively. By understanding and applying this formula, contact centers can ensure they meet their service level targets while maintaining operational efficiency.How NICE is Redefining Customer Experience
NICE offers the industry’s only unified AI platform for customer service automation. CXone Mpower revolutionizes how organizations automate customer service from start to finish—with channels, data, end-to-end workflows, and enterprise knowledge converging to improve customer experience at scale. With domain specific AI trained on the industry’s largest CX dataset, an open framework with endless integration possibilities, and a complete suite of advanced AI applications, CXone Mpower is one platform built for organizations of all sizes to deliver seamless customer service experiences, boost operational efficiency, and drive better outcomes.Omnichannel interaction routingDigital and Voice ChannelsAutomatic Call Distributor (ACD)Interactive Voice Response (IVR)Proactive Engagement
